Centers of Triangles

by

Hieu Huy Nguyen

The centroid is commonly referred to as the center of gravity for any matter. It's known is that point which is derictly centered within a uniform object matter. In this case, we explore the centroid of a triangle. Given a triangle ABC, we create line segments from the midpoints of the sides to the opposing vertex. The intersection of these line segments creates a point G, which is known as the centroid, "center of mass", or "center of gravity".

The center of mass idea indicates that the point is the absolute center which balances out the triangle. For this to be possible, means that the areas of the median triangles (interior) should have equal areas. The GSP file given here demonstrates the concept that areas of median triangles remain equal regardless of the shape of the triangle. (Instruction: click on any vertice of the triangle to modify it, and you'll notice that the areas calculated will remain equal).

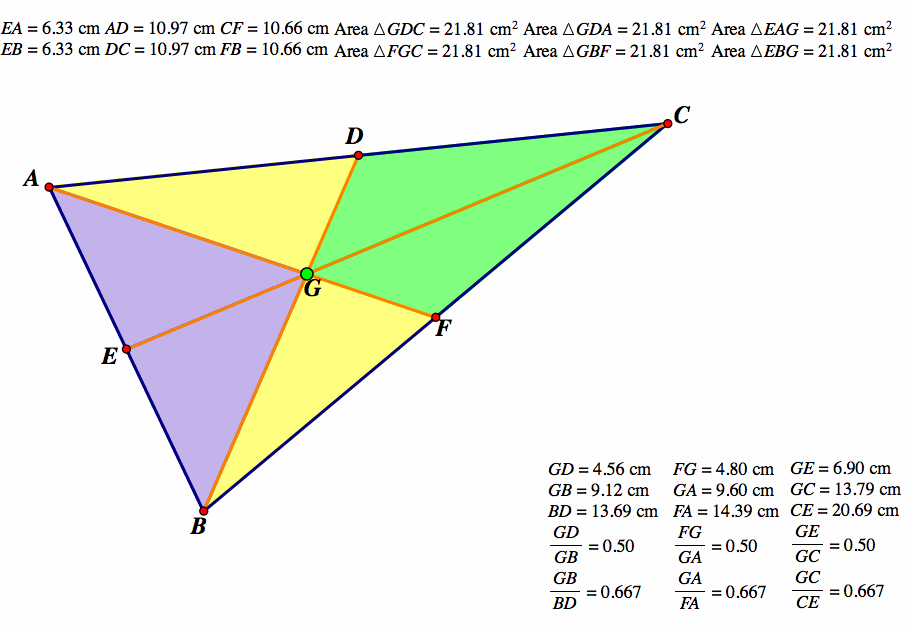
Because the areas of the interior median triangles remain equal, that means the center point G is the absolute point which marks the center of the body of the triangle. Also, we can recognize that the centroid is exactly 2/3 the distance from any midpoint to the opposite vertice. The animation also allows the distances to change, but the formula defining those distances remain the same.

Go back to Hieu's HOME PAGE

Go back to EMAT 6680 HOME PAGE